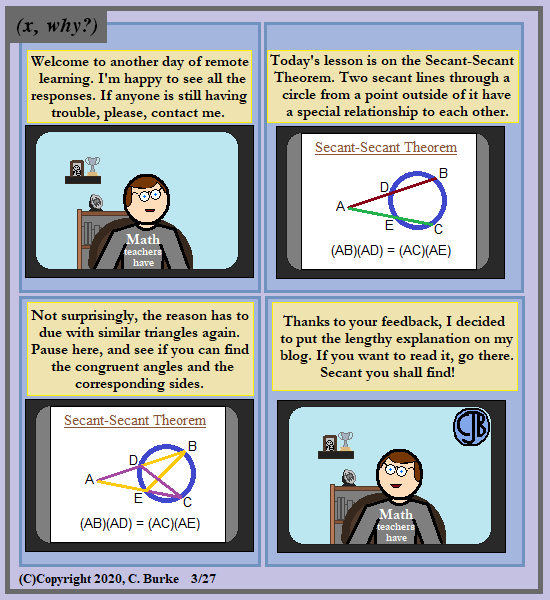
What do Math teachers have? Well, for one, T-shirts like that one!

Guesses to what my actual shirt says can be left in the comments.

So, if I reteach the same material year after year, I'm allowed to re-use the same puns, right? It's all new to them.

Since I said that I would explain on my blog, I guess I need to do that here.

As we see in Panel 2, above, we are Given Secant AB with point D on the circle, and AC with point E on the circle.
We want to prove that the products of these lengths are equal: (AB)(AD) = (AC)(AE)

If we draw chords CD and BE, we create triangles ABE and ACD, as shown in Panel 3.
Angles B and C both intercept the same arc, DE, and therefore they are congruent.
Angle A is congruent to itself because of the Reflexive Property.
Therefore, triangles ABE and ACD are similar.
If they are similar, then their corresponding sides are proportional.

So AB / AE = AC / AD
If we cross-multiply, we get: (AB)(AD) = (AC)(AE)

Or, in other words, "The whole line times the other part equals the other whole line times its outside part".

Actually, not very long, and could easily be included in an actual remote video, but not so much in a four- or six-panel comic page.